√70以上 1^2 2^2 3^2 .... n^2 = n(n 1)(2n 1)/6 brainly 871633

Try to make pairs of numbers from the set The first the last;(1) we will prove that the statement must be true for n = k 1Aug 15, 09 · 1^22^23^2n^2 We know that (x1)^3x ^3= 3x^23x1 Putting x=1,2n, we get 2^31^3=3(1)^23(1)1 3^32^3=3(2)^23(2)1 (n1)^3n^3=3(n)^23(n)1

5 The Sum Of Three Consecutive Integers Is 147 Which Equation Represents How To Find The Least Brainly Com

1^2 2^2 3^2 .... n^2 = n(n 1)(2n 1)/6 brainly

1^2 2^2 3^2 .... n^2 = n(n 1)(2n 1)/6 brainly-Solve for n 21/2n=3n16 Combine and Move all terms containing to the left side of the equation Tap for more steps Subtract from both sides of the equation Simplify the left side of the equation Tap for more steps To write as a fraction with a common denominator, multiply byThe sum of the first n squares, 1 2 2 2 n 2 = n(n1)(2n1)/6 For example, 1 2 2 2 10 2 =10×11×21/6=385 This result is usually proved by a method known as mathematical induction, and whereas it is a useful method for showing that a formula is true, it does not offer any insight into where the formula comes from

Simplify 3 2 N 6 9 2n 7 27 2n Brainly In

Nov 15, 11 · For induction, you have to prove the base case Then you assume your induction hypothesis, which in this case is 2 n >= n 2After that you want to prove that it is true for n 1, ie that 2 n1 >= (n1) 2You will use the induction hypothesis in the proof (the assumption that 2 n >= nM4maths previous todays puzzles The value of 2^n 2^(n1) / 2^(n1) 2^n is take any integer value say n=5 which gives the value of expression as 3/2Calcula las coordenadas de los puntos medios de los lados del triángulo de vértices , A(2,1) B(2,5) y C(2,3) AYUDA PORFA CON RESOLUCION EN UNA HOJA LUEGO LE TOMAN FOTO Indica si las siguientes sucesiones son progresivas aritméticas o geométricas y calcula su diferencia o su razón a) 4, 12, 36, 108, 324,

Simple and best practice solution for 2/3(1n)=1/2n equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve itWe simplify the equation to the form, which is simple to understand 1/2 (2n6)=5n12n Simplifying 05* (2n6)=5n12n Reorder the terms in parentheses (1n3)=5n12n Remove unnecessary parentheses 1n3=5n12n We move all terms containing n to the left and all other terms to the rightFor example 1/2 and 2/4 are equivalent, y/(y1) 2 and (y 2 y)/(y1) 3 are equivalent as well To calculate equivalent fraction , multiply the Numerator of each fraction, by its respective Multiplier

Dec 21,  · For each natural number \(n\), \(1^2 2^2 n^2 = \dfrac{n(n 1)(2n 1)}{6}\) One way of proving statements of this form uses the concept of an inductive set introduced in Preview Activity \(\PageIndex{2}\) The idea is to prove that if one natural number makes the open sentence true, then the next one also makes the open sentence true4 Applying other theorems about behavior of limits under arithmetic operations with sequences, we conclude that lim 1 2 q 1 1 4n 2 = 1 2·12 = 1 4 95 Let t1 = 1 and tn1 = (t2 n 2)/2tn for n ≥ 1 Assume that tn converges and find the limit2 See answers Brainly UserBrainly User SOLUTION Prove that 12 22 n2 = n(n1)(2n1)/6, for every positive integer n Proof I Prove that the equation holds for n = 1 If n = 1, then n(n1)(2n1)/6 = (1)(2)(3)/6 = 1 So, 12 = (1)(11)(2(1)1)/6

Ex 4 1 8 Prove 1 2 2 22 3 23 N 2n N 1 2n 1 2

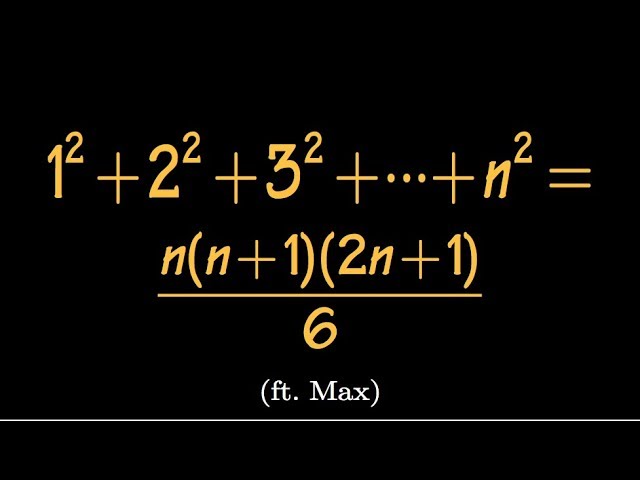
Find The Sum Of First N Squares Difference Equation Approach Ft Max Youtube

Statement P (n) is defined by 1 2 2 2 3 2 n 2 = n (n 1) (2n 1)/ 2 STEP 1 We first show that p (1) is true Left Side = 1 2 = 1 Right Side = 1 (1 1) (2*1 1)/ 6 = 1 Both sides of the statement are equal hence p (1) is true STEP 2 We now assume that p (k) is trueN2 2 The result is always n And since you are adding two numbers together, there are only (n1)/2 pairs that can be made from (n1) numbers So it is like (N1)/2 * N21 Pull out like factors 2n 2 = 2 • (n 1) Trying to factor by splitting the middle term 22 Factoring n 2 2n 3 The first term is, n 2 its coefficient is 1 The middle term is, 2n its coefficient is 2 The last term, "the constant", is 3 Step1 Multiply the coefficient of the first term by the constant 1 • 3 = 3

How To Prove That Math 2 4 6 8 2n N N 1 Math Quora

Prove That 1 2 3 2 3 4 3 4 5 N N 1 N 2 N N 1 N 2 N 3 4 Brainly In

1^22^23^2n^2=1/6 n(n1) (2n1)=Prove that $1^22^23^2(1)^{n1} n^2$=$(1)^{n1}\frac{ n(n1)}{2}$ whenever n is a positive integer using mathematical induction Ask Question Asked 7 years, 7 months agoFor the proof, we will count the number of dots in T(n) but, instead of summing the numbers 1, 2, 3, etc up to n we will find the total using only one multiplication and one division!

5 2n 3 25 N 2 125 N 1 2 3 Brainly In

Mathematical Induction Prove That 1 1 2 3 1 2 3 4 N N 3 4 N 1 N 2 Youtube

SOLUTION use mathematical induction to prove that 1^2 2^2 3^2 n^2 = n(n1)(2n1)/6 for all positive integral values of n Algebra > Equations > SOLUTION use mathematical induction to prove that 1^2 2^2 3^2 n^2 = n(n1)(2n1)/6 for all positive integral values of n Log OnApr 12, 21 · An efficient approach is to find the 2^(n1) and subtract 1 from it since we know that 2^n can be written as 2 n = ( 2 0 2 1 2 2 2 3 2 4 2 n1) 1 Below is the implementation of above approachAug 18, 17 · #= 1^2 2^2 3^2 The #N# th term would be given by #(1)^(N1)N^2# , and the finite sum at the #N# th term would be found as follows If this series were not alternating, the sum would have been

Solved 5 A N 1 6 An Vn 7 1 3 N 8 10 N Chegg Com

Quantum Numbers For The First Four Shells Video Khan Academy

Apr , 21 · Time Complexity O(n) Another approach Using formula to find sum of series 1 2 3 2 5 2 7 2 (2*n 1) 2 = (n * (2 * n 1) * (2 * n 1)) / 3 Please refer sum of squares of even and odd numbers for proofJun 27, 17 · #"using the method of "color(blue)"proof by induction"# #"this involves the following steps "# #• " prove true for some value, say n = 1"# #• " assume the result is true for n = k"#In Exercises 115 use mathematical induction to establish the formula for n 1 1 12 22 32 n2 = n(n 1)(2n 1) 6 Proof For n = 1, the statement reduces to 12 = 1 2 3 6 and is obviously true Assuming the statement is true for n = k 12 22 32 k2 = k(k 1)(2k 1) 6;

1 2 2 2 2 3 2 3 N 2 N N 1 2 N 1 2 Youtube

3 9 N 1 9 3 2n 3 3 3 2n 3 9 N 1 2 Simplify Brainly In

MAT V1102 – 004 Solutions page 2 of 7 8 Since ex is a strictly increasing function, e1/n ≤ e for all n ≥ 1 Hence, we have e1/n n3/2 ≤ e n3/2 Since P en−3/2 converges (it's a pseries with p = 3/2 > 1), the comparison test implies that P e1/nn−3/2 also converges 9 If f(n) = (n2)(n3) (n1)3 then f′(n) = (2n5)(n1)3 − 3(n2 5n6)(n1)2 (n1)6 = − n2 8n13My question $(n1)^2(n2)^2(n3)^2(2n)^2= \frac{n(2n1)(7n1)}{6}$ My workings LHS=$2^2$ =$4$ RHS= $\frac{24}{6} =4 $ $(k1)^2(k2)^2(k3)^2(2k)^2We now have the relation true for n = 1 and if the relation is true for any n greater than 1, it it also true for n 1 This proves that for all values of n 1^2 2^2 3^2 n^2 = 1/6 n(n1

2 N 6 M 1 10 M N 15 M N 2 Divided By 4 M 3 2m N 25 M 1 Brainly In

Question 4 Of 8 Consider The Recursive Function Of An Arithmetic Sequence Below F 1 3 F N F N Brainly Com

The value of n in the equation is 1 Stepbystep explanation The equation is (2n 4) 6 = 9 4(2n 1) At first we must do that 1 Open the brackets in both sides 2 Add like terms 3 Solve to find n LHS ∵ (2n 4) 6 = n 2 6 ∴ (2n 4) 6 = n 4 RHS ∵ 9 4(2n 1) = 9 8n 4 ∴ 9 4(2n 1) = 8n 5As I know the formula for adding 1,2,3n is given by n(n1)/2 Comparing to above formula if we want to calculate sum up to n1 , using the above formula we get n1(n11)/2 That is n(n1)/2 Thus the required formula is n(n1)/2Buktikan bahwa (n1) 2

Determine Whether The Series Is Convergent Or Divergent By Expressing Sn As A Telescopic Sum As In Brainly Com

Question No 8 Please Solve Brainly In

O(2^(n1)) is the same as O(2 * 2^n), and you can always pull out constant factors, so it is the same as O(2^n) However, constant factors are the only thing you can pull out 2^(2n) can be expressed as (2^n)(2^n), and 2^n isn't a constant So, the answer to your questions are yes and noI have wondered how the closed form for the sum of squares for the first n natural numbers was derived Given the formula for the sum 1^22^2n^2= n(n1)(2n1)/6 I learned to prove its correctness using mathematical induction However, I neverN 4 = 8n 5 Next step is to move everything to it's appropriate places and make the variable equal to something, in other words, solve for n n 4 = 8n 59n = 9 Since the variable is negative and has a number of nine, we divide the whole equation by 9 in order to make it positive and leave "n" by itself 9n = 9 n = 1 Your answer

What Is The Value Of N In The Equation 1 2 N 4 3 3 2n 3 N 0 N 2 N 4 N 6 Brainly Com

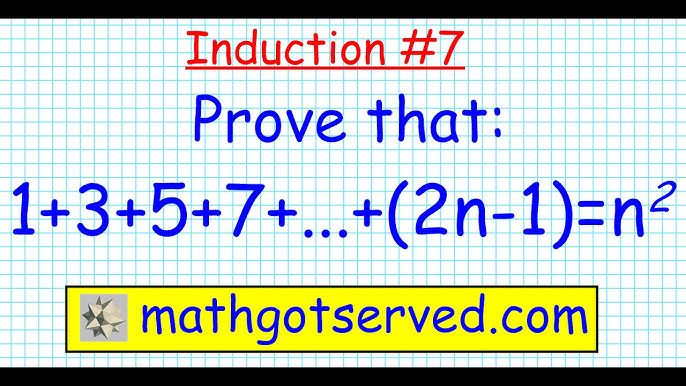
7 Proof By Induction 1 3 5 7 2n 1 N 2 Discrete Prove All N In N Induction Mathgotserved Youtube

To do this, we will fit two copies of a triangle of dots together, one red and an upsidedown copy in green Eg T(4)=1234 =The second the one before last It means n1 1;Conclusion By the principle of induction, it follows that is true for all n 2Z 8 Prove that f n (3=2)n 2 for all n 2Z Proof We will show that for all n 2Z , f n (3=2)n 2 Base cases When n = 1, the left side of is f 1 = 1, and the right side is (3=2) 1 = 2=3, so holds for n = 1 When n =

Solve For N 2 3 1 N 1 2n Brainly Com

Use The Bank Of Numbers Below Which Number Makes Each Equation Below True 72 6n 8 N 2 N Brainly Com

Sep 14, 10 · 1^2 2^2 3^2 2^n = 2^(n1) This makes absolutely no sense I mean, look at it for a second firstly you failed to notice the pattern correctly since 2^n means 2^12^22^3 instead of what is shownAnswer to Prove that 1^2 2^2 3^2 n^2 = n(n 1)(2n 1)/6 for n greaterthanorequalto 1 Prove that 1^2 3^2 5^2Solve for n 32(n4)>1 Simplify Tap for more steps Simplify each term Tap for more steps Apply the distributive property Multiply by Add and Move all terms not containing to the right side of the inequality Tap for more steps Subtract from both sides of the inequality

For A Proof By Induction Of The Math Statement Below Identify The Correct Step For Proving The Brainly Com

1 2 3 4 N 1 2 N N 1 Prove With Mathematical Induction Brainly In

# Python Program to calculate Sum of Series 1²2²3²n² def sum_of_square_series(number) total = 0 total = (number * (number 1) * (2 * number 1)) / 6 for i in range(1, number 1) if(i != number) print("%d^2 " %i, end = ' ') else print("{0}^2 = {1}"format(i, total)) num = int(input("Please Enter any Positive Number ")) sum_of_square_series(num)Nov 17, 13 · "1^22^23^2n^2=n(n1)(2n1)/6を証明しました多分これより簡単な証明はないはず!2 Metode pembuktian untuk proposisi yang berkaitan dengan bilangan bulat adalah induksi matematik Contoh 1 Buktikan bahwa jumlah n bilangan bilangan bulat positif pertama adalah n(n 1)/2 2 Buktikan bahwa jumlah n buah bilangan ganjil positif pertama adalah n2 Rinaldi Munir/IF21 Matematika Diskrit

5 The Sum Of Three Consecutive Integers Is 147 Which Equation Represents How To Find The Least Brainly Com

What Is The Value Of N In The Equation Brainly Com

Answer to Prove1 2 4 6 2n = n(n 1)2 3 6 9 3n = (3n(n 1))/23 2 5 8 (3n 1) = (n(3n 1))4 P 1 n=1 n2 41 Answer Let a n = n2=(n4 1) Since n4 1 >n4, we have 1 n41 < 1 n4, so a n = n 2 n4 1 n n4 1 n2 therefore 0

5 N 3 6 5 N 1 9 5 N 5 N 2 2 Brainly In

Ex 4 1 6 1 2 2 3 3 4 N N 1 N N 1 N 2 3

Find The Value Of N In Each Of The Following A N 4 1 2 N 3 1 Brainly In

If Sn 1 3 6 10 N N 1 2 Then Sn Is Brainly In

Prove That 2n N 1 3 5 2n 1 2 Nplzzzzzzz Ans Plzzzzzz Brainly In

Prove That 2n 2 N N 1 3 5 2n 1 Brainly In

What Is The Summation Of The Series 1 3 6 10 15 Quora

How To Solve This Recurrence T N 2t N 2 N Log N Mathematics Stack Exchange

Find N If 2n 1 P N 1 2n 1pn 3 5 Brainly In

Identifying And Graphing Sequences Lesson 4 1 Complete The Table And State The Domain And Range For Brainly Com

In The Sequence Of Sets 1 2 3 4 5 6 7 8 9 10 And So On What Is The Sum Of Elements In The 50th Set Quora

10 Best Math Apps For Android For Better Math Skills Android Authority

Simplify 3 2 N 6 9 2n 7 27 2n Brainly In

Prove By Pmi That 1 2 2 3 3 4 N N 1 N N 1 N 2 3 N In N Youtube

How To Find N Value In 2 2n 1 1 8 N 3 Find Tha Value Of N Brainly In

2 5 8 3n 1 N 3n 1 2 Brainly In

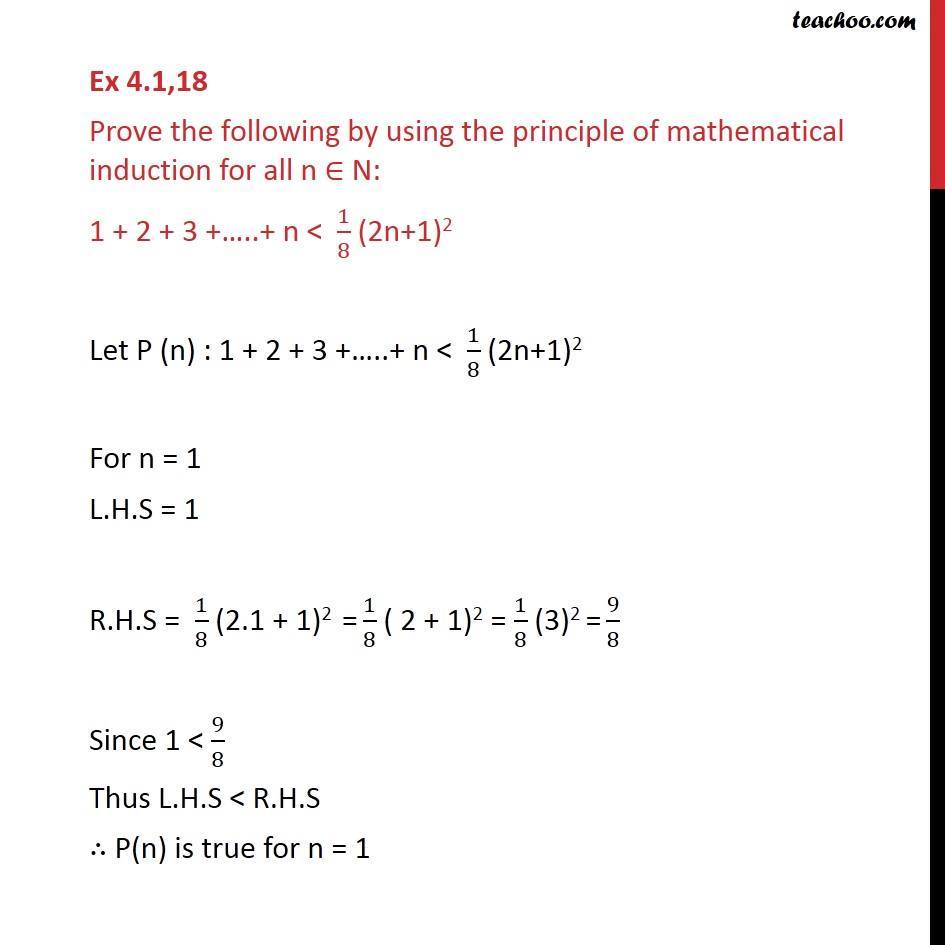
Ex 4 1 18 Prove 1 2 3 N 1 8 2n 1 2 Induction

A Sequence Is Shown 3 6 12 24 48 Which Function F N Represents The Nth Term Of The Brainly Com

9n 2 3 N 2 2 27n 3m 2 3 1 729 Then Prove M N 2 Brainly In

Pls Help Me Plssssssssssssss Brainly Com

I Need Help With Questions 4 And 5 Someone Please Help Brainly Com

Prove 2 6 10 14 To N Factors 2 N N N 1 N 2 To N Factors Brainly In

Op 4 6 8 Brainly Richard Hol

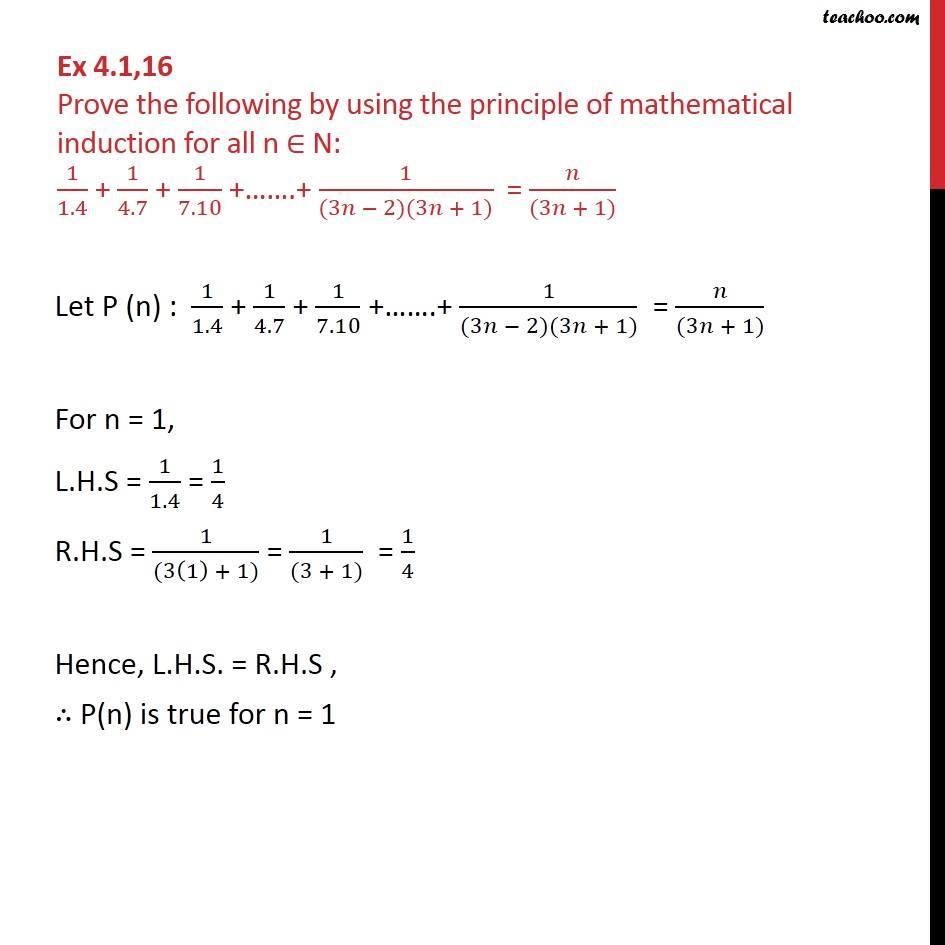
Ex 4 1 16 Prove 1 1 4 1 4 7 1 3n 2 3n 1 N 3n 1

By Mathematical Induction Prove That 1 2 3 N N N 1 2n 1 6 Brainly In

I Really Need Helppppp Write A Paragraph Proof For The Following Conjecture Given M 1 M 2 Brainly Com

Identify The Recursive Formula For The Sequence Given By The Explicit Formula An 41n 1 A Brainly Com

By Mathematical Induction Prove That 1 2 3 N N N 1 2n 1 6 Brainly In

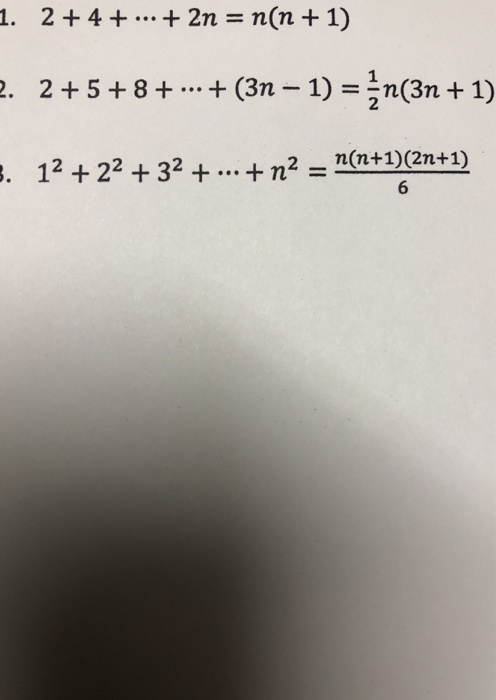
Solved 1 2 4 2n N N 1 2 5 8 3n 1 N 3n 1 12 Chegg Com

1 3 5 2n 1 N 2n 1 2n 1 3mathematical Inductionplzzz Solve This No Spam Brainly In

Consider The Equation Below 57 31 14n 5 Select The Equation That Has The Same Solution A Brainly Com

70以上 5 2n Sequence 5 2n Sequence

Examine The Behaviour Of Infinite Series Of Positive Terms 1 2 1 3 2 4 1 3 5 2 4 Brainly In

What Is The Summation Of The Series 1 3 6 10 15 Quora

Simplify Each Expression And Then Arrange Them In Increasing Order Based On The Coefficient Of N2 Brainly Com

Evaluate G N 5 If G X X 2 6 2x Brainly Com

An Arithmetic Sequence A Starts 2 5 1 Use Your Definition To Make A Table Of Values For A N Brainly Com

If N 2 Is Even Then N Is Even Chilimath

Simplify Each Expression And Then Arrange Them In Increasing Order Based On The Coefficient Of N 2 Brainly Com

Simplify 16 2 N 1 4 2 N 16 2 N 2 2 2 N 2 Brainly In

I Will Give Brainliest Can You Please Put The Answers By The Questions Thx I You See 69 For Brainly Com

Given A Sequence Is Defined By The Explicit Definition Latex T N N 2 Nt N N 2 N Find The 4th Brainly Com

What Is The Summation Of The Series 1 3 6 10 15 Quora

N N 1 2 1 N 2 3 Brainly In

2n 3 n 2 N 10n 2 Brainly Com

N N 1 N 2 N 3 N 4 N 5 Find The Average Value Brainly In

G N 4n 5 F N N 3 3n 2 Find G F N Brainly Com

N N 1 2 1 N 2 3 Brainly In

Show That 2ncn 2 N 1 3 5 2n 1 N Brainly In

Simplify 2 N 2 N 1 2 N 1 2 N Brainly In

I Need Help Asap Will Give Brainliestwhich Shows The Series 0 2 6 12 Written In Sigma Brainly Com

The Sum Of The Series 2 3 8 9 26 27 80 81 To N Terms

In The Geometric Pattern Below N Represents The Number Of Blocks In The Bottom Row Of Each Brainly Com

3 Principle Of Mathematical Induction Prove Proof 1 5 9 4n 3 N 2n 1 Youtube

N N 1 2 1 N 2 3 Brainly In

Simplify The Expression 6 N 2 N 1 Brainly Com

The Solution Set To 6 2n Gt 12 Is N Gt 3 Which Are Correct Representations Of This Solution Brainly Com

1 2 2 4 3 8 N 2n 2 N 2 2 N Brainly Co Id

If F N N 1 2 3n Which Statement Is True 1 F 3 2 2 F 2 3 3 F 2 15 4 F 15 Brainly Com

Find 4 1 N 7 2 N 10 3 N Upto N Terms Class 10 Brainly In

Lim N Oo N 1 N 2 N 3 3n N 2n 1 N Is Equa

Simplify X 2n 3 X 2n 1 N 2 Upon X 3 2n 1 X N 2n 1 Brainly In

Y X 3 X X Elements Of Natural Number Re Write In Number Brainly Com

Can Someone Please Help Me Brainly Com

Prove By The Method Of Induction For All N Belongs To N 1 2 2 2 3 2 N 2 N N 1 2n 1 6 Brainly In

Which Verbal Expression Represents 2 N 6 Brainly Com

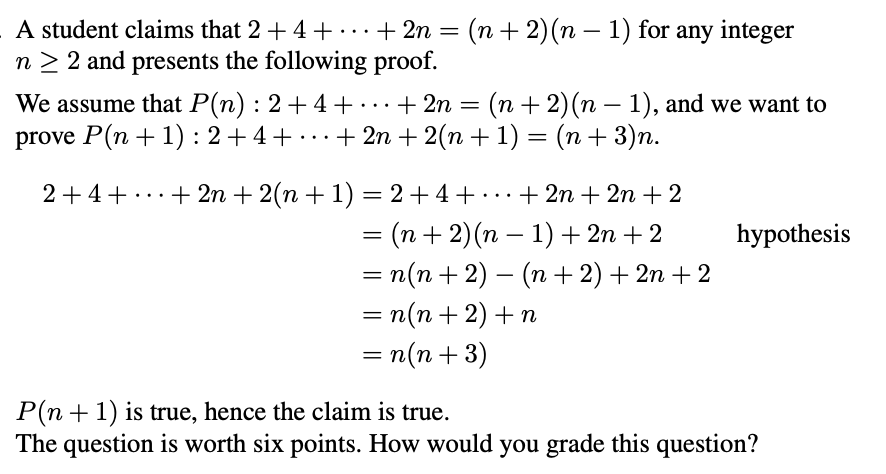
Solved A Student Claims That 2 4 2n N 2 Chegg Com

For Each Sequence Find The First 4 Terms And The 10th Term A 12 N B 5 2n C N 2 3 D Brainly Com

Nth Term Of An Arithmetic Progression Video Khan Academy

Which Equation Finds The Volume Of A Cube With A Side Length Of 2n 6 Units A 2n 6 3 8n 18 Cubic Brainly Com

Question1 1 1 2 1 1 2 3 1 1 2 3 N 2n N 1 Brainly In

Solves This Qustion According To Principale Of Mathematical Induction Brainly In

Ex 4 1 4 Prove 1 2 3 2 3 4 N N 1 N 2 N N 1

Ex 4 1 11 Prove 1 1 2 3 1 2 3 4 1 3 4 5 1 N N 1 N 2

Which Of The Following Recursive Formulas Represents The Same Geometric Sequence As The Formula Brainly Com

I Need Help With 1 5 Brainly Com